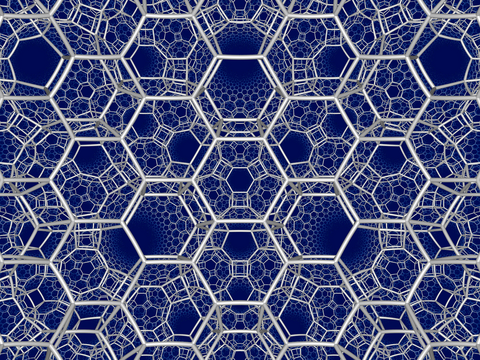
| Order-6 cubic honeycomb | |
|---|---|
 Perspective projection view within Poincaré disk model | |
| Type | Hyperbolic regular honeycomb Paracompact uniform honeycomb |
| Schläfli symbol | {4,3,6} {4,3} |
| Coxeter diagram | |
| Cells | {4,3} |
| Faces | square {4} |
| Edge figure | hexagon {6} |
| Vertex figure |   triangular tiling |
| Coxeter group | , , |
| Dual | Order-4 hexagonal tiling honeycomb |
| Properties | Regular, quasiregular |
The order-6 cubic honeycomb is a paracompact regular space-filling tessellation (or honeycomb) in hyperbolic 3-space. It is paracompact because it has vertex figures composed of an infinite number of facets, with all vertices as ideal points at infinity. With Schläfli symbol {4,3,6}, the honeycomb has six ideal cubes meeting along each edge. Its vertex figure is an infinite triangular tiling. Its dual is the order-4 hexagonal tiling honeycomb.
A geometric honeycomb is a space-filling of polyhedral or higher-dimensional cells, so that there are no gaps. It is an example of the more general mathematical tiling or tessellation in any number of dimensions.
Honeycombs are usually constructed in ordinary Euclidean ("flat") space, like the convex uniform honeycombs. They may also be constructed in non-Euclidean spaces, such as hyperbolic uniform honeycombs. Any finite uniform polytope can be projected to its circumsphere to form a uniform honeycomb in spherical space.
Images
 One cell viewed outside of the Poincaré sphere model |
 The order-6 cubic honeycomb is analogous to the 2D hyperbolic infinite-order square tiling, {4,∞} with square faces. All vertices are on the ideal surface. |
Symmetry
A half-symmetry construction of the order-6 cubic honeycomb exists as {4,3}, with two alternating types (colors) of cubic cells. This construction has Coxeter-Dynkin diagram ![]()
![]()
![]()
![]()
![]()
![]()
![]() ↔
↔ ![]()
![]()
![]()
![]()
![]() .
.
Another lower-symmetry construction, , of index 6, exists with a non-simplex fundamental domain, with Coxeter-Dynkin diagram ![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
This honeycomb contains ![]()
![]()
![]()
![]()
![]() that tile 2-hypercycle surfaces, similar to the paracompact order-3 apeirogonal tiling,
that tile 2-hypercycle surfaces, similar to the paracompact order-3 apeirogonal tiling, ![]()
![]()
![]()
![]()
![]() :
:
Related polytopes and honeycombs
The order-6 cubic honeycomb is a regular hyperbolic honeycomb in 3-space, and one of 11 which are paracompact.
| 11 paracompact regular honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
 {6,3,3} |
 {6,3,4} |
 {6,3,5} |
 {6,3,6} |
 {4,4,3} |
 {4,4,4} | ||||||
 {3,3,6} |
 {4,3,6} |
 {5,3,6} |
 {3,6,3} |
 {3,4,4} | |||||||
It has a related alternation honeycomb, represented by ![]()
![]()
![]()
![]()
![]()
![]()
![]() ↔
↔ ![]()
![]()
![]()
![]()
![]() . This alternated form has hexagonal tiling and tetrahedron cells.
. This alternated form has hexagonal tiling and tetrahedron cells.
There are fifteen uniform honeycombs in the Coxeter group family, including the order-6 cubic honeycomb itself.
| family honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| {6,3,4} | r{6,3,4} | t{6,3,4} | rr{6,3,4} | t0,3{6,3,4} | tr{6,3,4} | t0,1,3{6,3,4} | t0,1,2,3{6,3,4} | ||||

|

|

|

|

|

|

|

| ||||

|

|

|

|

|

|

| |||||
| {4,3,6} | r{4,3,6} | t{4,3,6} | rr{4,3,6} | 2t{4,3,6} | tr{4,3,6} | t0,1,3{4,3,6} | t0,1,2,3{4,3,6} | ||||
The order-6 cubic honeycomb is part of a sequence of regular polychora and honeycombs with cubic cells.
| {4,3,p} regular honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Space | S | E | H | ||||||||
| Form | Finite | Affine | Compact | Paracompact | Noncompact | ||||||
| Name |
{4,3,3} |
{4,3,4} |
{4,3,5} |
{4,3,6} |
{4,3,7} |
{4,3,8} |
... {4,3,∞} | ||||
| Image | 
|

|

|

|

|
|

| ||||
| Vertex figure |
 {3,3} |
 {3,4} |
 {3,5} |
 {3,6} |
 {3,7} |
 {3,8} |
 {3,∞} | ||||
It is also part of a sequence of honeycombs with triangular tiling vertex figures.
| Form | Paracompact | Noncompact | |||||
|---|---|---|---|---|---|---|---|
| Name | {3,3,6} | {4,3,6} | {5,3,6} | {6,3,6} | {7,3,6} | {8,3,6} | ... {∞,3,6} |
| Image | 
|

|

|

|

|

|

|
| Cells | {3,3} |
{4,3} |
{5,3} |
{6,3} |
{7,3} |
{8,3} |
{∞,3} |
Rectified order-6 cubic honeycomb
| Rectified order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | r{4,3,6} or t1{4,3,6} |
| Coxeter diagrams | |
| Cells | r{3,4} {3,6} |
| Faces | triangle {3} square {4} |
| Vertex figure |  hexagonal prism |
| Coxeter groups | , , , , |
| Properties | Vertex-transitive, edge-transitive |
The rectified order-6 cubic honeycomb, r{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has cuboctahedral and triangular tiling facets, with a hexagonal prism vertex figure.
has cuboctahedral and triangular tiling facets, with a hexagonal prism vertex figure.
It is similar to the 2D hyperbolic tetraapeirogonal tiling, r{4,∞}, ![]()
![]()
![]()
![]()
![]() alternating apeirogonal and square faces:
alternating apeirogonal and square faces:
| Space | H | ||||||
|---|---|---|---|---|---|---|---|
| Form | Paracompact | Noncompact | |||||
| Name | r{3,3,6} |
r{4,3,6} |
r{5,3,6} |
r{6,3,6} |
r{7,3,6} |
... r{∞,3,6} | |
| Image | 
|

|

|

|
|||
| Cells {3,6} |
r{3,3} |
r{4,3} |
r{5,3} |
r{6,3} |
r{7,3} |
r{∞,3} | |
Truncated order-6 cubic honeycomb
| Truncated order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | t{4,3,6} or t0,1{4,3,6} |
| Coxeter diagrams | |
| Cells | t{4,3} {3,6} |
| Faces | triangle {3} octagon {8} |
| Vertex figure |  hexagonal pyramid |
| Coxeter groups | , , |
| Properties | Vertex-transitive |
The truncated order-6 cubic honeycomb, t{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated cube and triangular tiling facets, with a hexagonal pyramid vertex figure.
has truncated cube and triangular tiling facets, with a hexagonal pyramid vertex figure.
It is similar to the 2D hyperbolic truncated infinite-order square tiling, t{4,∞}, ![]()
![]()
![]()
![]()
![]() with apeirogonal and octagonal (truncated square) faces:
with apeirogonal and octagonal (truncated square) faces:
Bitruncated order-6 cubic honeycomb
The bitruncated order-6 cubic honeycomb is the same as the bitruncated order-4 hexagonal tiling honeycomb.
Cantellated order-6 cubic honeycomb
| Cantellated order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | rr{4,3,6} or t0,2{4,3,6} |
| Coxeter diagrams | |
| Cells | rr{4,3} r{3,6} {}x{6} |
| Faces | triangle {3} square {4} hexagon {6} |
| Vertex figure |  wedge |
| Coxeter groups | , , |
| Properties | Vertex-transitive |
The cantellated order-6 cubic honeycomb, rr{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has rhombicuboctahedron, trihexagonal tiling, and hexagonal prism facets, with a wedge vertex figure.
has rhombicuboctahedron, trihexagonal tiling, and hexagonal prism facets, with a wedge vertex figure.
Cantitruncated order-6 cubic honeycomb
| Cantitruncated order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | tr{4,3,6} or t0,1,2{4,3,6} |
| Coxeter diagrams | |
| Cells | tr{4,3} t{3,6} {}x{6} |
| Faces | square {4} hexagon {6} octagon {8} |
| Vertex figure |  mirrored sphenoid |
| Coxeter groups | , , |
| Properties | Vertex-transitive |
The cantitruncated order-6 cubic honeycomb, tr{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated cuboctahedron, hexagonal tiling, and hexagonal prism facets, with a mirrored sphenoid vertex figure.
has truncated cuboctahedron, hexagonal tiling, and hexagonal prism facets, with a mirrored sphenoid vertex figure.
Runcinated order-6 cubic honeycomb
The runcinated order-6 cubic honeycomb is the same as the runcinated order-4 hexagonal tiling honeycomb.
Runcitruncated order-6 cubic honeycomb
| Cantellated order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | t0,1,3{4,3,6} |
| Coxeter diagrams | |
| Cells | t{4,3} rr{3,6} {}x{6} {}x{8} |
| Faces | triangle {3} square {4} hexagon {6} octagon {8} |
| Vertex figure | isosceles-trapezoidal pyramid |
| Coxeter groups | , |
| Properties | Vertex-transitive |
The runcitruncated order-6 cubic honeycomb, rr{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated cube, rhombitrihexagonal tiling, hexagonal prism, and octagonal prism facets, with an isosceles-trapezoidal pyramid vertex figure.
has truncated cube, rhombitrihexagonal tiling, hexagonal prism, and octagonal prism facets, with an isosceles-trapezoidal pyramid vertex figure.
Runcicantellated order-6 cubic honeycomb
The runcicantellated order-6 cubic honeycomb is the same as the runcitruncated order-4 hexagonal tiling honeycomb.
Omnitruncated order-6 cubic honeycomb
The omnitruncated order-6 cubic honeycomb is the same as the omnitruncated order-4 hexagonal tiling honeycomb.
Alternated order-6 cubic honeycomb
| Alternated order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb Semiregular honeycomb |
| Schläfli symbol | h{4,3,6} |
| Coxeter diagram | |
| Cells | {3,3} {3,6} |
| Faces | triangle {3} |
| Vertex figure |  trihexagonal tiling |
| Coxeter group | , , |
| Properties | Vertex-transitive, edge-transitive, quasiregular |
In three-dimensional hyperbolic geometry, the alternated order-6 hexagonal tiling honeycomb is a uniform compact space-filling tessellation (or honeycomb). As an alternation, with Schläfli symbol h{4,3,6} and Coxeter-Dynkin diagram ![]()
![]()
![]()
![]()
![]()
![]()
![]() or
or ![]()
![]()
![]()
![]()
![]() , it can be considered a quasiregular honeycomb, alternating triangular tilings and tetrahedra around each vertex in a trihexagonal tiling vertex figure.
, it can be considered a quasiregular honeycomb, alternating triangular tilings and tetrahedra around each vertex in a trihexagonal tiling vertex figure.
Symmetry
A half-symmetry construction from the form {4,3} exists, with two alternating types (colors) of triangular tiling cells. This form has Coxeter-Dynkin diagram ![]()
![]()
![]()
![]()
![]()
![]()
![]() ↔
↔ ![]()
![]()
![]()
![]()
![]() . Another lower-symmetry form of index 6, , exists with a non-simplex fundamental domain, with Coxeter-Dynkin diagram
. Another lower-symmetry form of index 6, , exists with a non-simplex fundamental domain, with Coxeter-Dynkin diagram ![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Related honeycombs
The alternated order-6 cubic honeycomb is part of a series of quasiregular polychora and honeycombs.
| Quasiregular polychora and honeycombs: h{4,p,q} | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Space | Finite | Affine | Compact | Paracompact | |||||||
| Schläfli symbol |
h{4,3,3} | h{4,3,4} | h{4,3,5} | h{4,3,6} | h{4,4,3} | h{4,4,4} | |||||
| Coxeter diagram |
|||||||||||
| Image | 
|

|

|

| |||||||
| Vertex figure r{p,3} |
 |
 |
 |
 |
 |
 | |||||
It also has 3 related forms: the cantic order-6 cubic honeycomb, h2{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() ; the runcic order-6 cubic honeycomb, h3{4,3,6},
; the runcic order-6 cubic honeycomb, h3{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() ; and the runcicantic order-6 cubic honeycomb, h2,3{4,3,6},
; and the runcicantic order-6 cubic honeycomb, h2,3{4,3,6}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Cantic order-6 cubic honeycomb
| Cantic order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | h2{4,3,6} |
| Coxeter diagram | |
| Cells | t{3,3} r{6,3} t{3,6} |
| Faces | triangle {3} hexagon {6} |
| Vertex figure |  rectangular pyramid |
| Coxeter group | , , |
| Properties | Vertex-transitive |
The cantic order-6 cubic honeycomb is a uniform compact space-filling tessellation (or honeycomb) with Schläfli symbol h2{4,3,6}. It is composed of truncated tetrahedron, trihexagonal tiling, and hexagonal tiling facets, with a rectangular pyramid vertex figure.
Runcic order-6 cubic honeycomb
| Runcic order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | h3{4,3,6} |
| Coxeter diagram | |
| Cells | {3,3} {6,3} rr{6,3} |
| Faces | triangle {3} square {4} hexagon {6} |
| Vertex figure |  triangular cupola |
| Coxeter group | , |
| Properties | Vertex-transitive |
The runcic order-6 cubic honeycomb is a uniform compact space-filling tessellation (or honeycomb) with Schläfli symbol h3{4,3,6}. It is composed of tetrahedron, hexagonal tiling, and rhombitrihexagonal tiling facets, with a triangular cupola vertex figure.
Runcicantic order-6 cubic honeycomb
| Runcicantic order-6 cubic honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | h2,3{4,3,6} |
| Coxeter diagram | |
| Cells | t{6,3} tr{6,3} t{3,3} |
| Faces | triangle {3} square {4} hexagon {6} dodecagon {12} |
| Vertex figure | 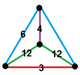 mirrored sphenoid |
| Coxeter group | , |
| Properties | Vertex-transitive |
The runcicantic order-6 cubic honeycomb is a uniform compact space-filling tessellation (or honeycomb), with Schläfli symbol h2,3{4,3,6}. It is composed of truncated hexagonal tiling, truncated trihexagonal tiling, and truncated tetrahedron facets, with a mirrored sphenoid vertex figure.
See also
- Convex uniform honeycombs in hyperbolic space
- Regular tessellations of hyperbolic 3-space
- Paracompact uniform honeycombs
References
- Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Tables I and II: Regular polytopes and honeycombs, pp. 294–296)
- The Beauty of Geometry: Twelve Essays (1999), Dover Publications, LCCN 99-35678, ISBN 0-486-40919-8 (Chapter 10, Regular Honeycombs in Hyperbolic Space) Table III
- Jeffrey R. Weeks The Shape of Space, 2nd edition ISBN 0-8247-0709-5 (Chapter 16-17: Geometries on Three-manifolds I, II)
- Norman Johnson Uniform Polytopes, Manuscript
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
- N.W. Johnson: Geometries and Transformations, (2018) Chapter 13: Hyperbolic Coxeter groups
 ,
,  ,
,

 ,
,  ,
,